Analysis and Design of Building Components (Part 3)

COLUMN (C-7):-

Assume column size = 10″ x 15″ = 150 sq. inch

a) Load Calculation:-

Load from F. B – A2,A3 = = 15.19 kip

Load from F. B – A3,A4 = = 8.25 kip

Load from F. B – A3,D3 = = 15.95 kip

Self weight of Column = x 150 x (10 – ) = 1.40 k

Factored load = 1.2 x 1.40 + 15.19 + 8.25 + 15.95

= 41.07 kip

Total column load for 6 – stored = 6 x 41.07 = 246.42 kip

b) Check for size:-

P_u = 243.11 (kip) (story -1) / ρ_g = 0.02

P_u = A_g [ .85f′c (1- ρ_g) + ρ_gf_y]

Or, 246.42 = 0.80 x 0.65 x Ag [0.85 x 3 (1- 0.02) + .02 x 40]

A_g = 143.64 inch

Size of column = 10″ x 15″

Provided size = 150 in

c) Calculation of steel:-

P_u = [0.85 x f′c (A_g – A_st) + A_st f_y]

Or, 150 = .80 x .65 x [.85 x 3 x (150- A_st) + A_st x 40]

A_st = 2.27 in

Use # 7 bars, Ap = 2.40 in

Tie bar:-

Use # 3 bar @ as Tie

S = 16 x Main bar dia = 16 x = 14″ c / c

S = 48 x Tie bar dia = 48 x = 18″ c / c

S = Minimum width of column = 10″ c /c

We use # 3 bar @ 10″ c / c

COLUMN (C-8):-

Assume column size = 10″ x 24″

a) Load Calculation:-

Load from F. B – B2,B3 = = 24.08 kip

Load from F. B – B3,B4 = = 13.08 kip

Load from F. B – B3,C3 = = 8.11 kip

Load from F. B – D3,B3 = = 3.12 kip

Self weight of Column = x 150 x (10 – ) = 2.25 k

Factored load = 24.08 + 13.08 + 8.11 + 1.2 x 2.25+20 k

= 67.97 kip

Total column load for 6 – story = 67.97 x 6 = 407.82 kip

b) Check for size:-

P_u = 407.82 (kip)

P_u = A_g [ .85f’c (1- ρ_g) + ρ_gf_y]

Or, 407.82 = 0.80 x 0.65 x A_g [0.85 x 3 (1- .02) + .02 x 40]

A_g = 237.80 in

Size of column = 10″ x 24″

Provided size = 240 in

So, Ok

c) Calculation of steel:-

P_u = [0.85 f′c (A_g – A_st) + A_st f_y]

Or, 407.82 = .80 x .65 x [.85 x 3 x (240- A_st) + A_stx 40]

A_st = 4.59 in

Use 8 # 7 bars st, Ap = 4.80 in

Tie bar:

Use # 3 bar as Tie

S = 16 x = 10″ c / c

S = 48 x = 18″ c / c

S = Minimum width of column = 10″ c / c

We use # 3 bar @ 10″ c / c

COLUMN (C-9):-

Assume column size = 10″ x 15″ = 150 sq. inch

a) Load Calculation:

Load from F. B – B3, C3 = = 7.81 kip

Load from F. B – C2, C3 = = 6.81 kip

Load from F. B – C3, C4 = = 3.06 kip

Load from stair Beam = = 19.38 kip

Self weight of Column = x 150 x (10 – )/1000 = 1.97 k

Factored load = 1.2 x 1.97 + 7.81 + 3.06 + 19.38 +20

= 59.42 kip

Total column load for 6. story = 6 x 59.42= 356.54 kip

b) Check for size:-

P_u= 116.232 kip

P_u = Ag [ .85f′c (1- ρ_g) + ρ_gf_y]

Or, 356.54 = 0.80 x .65 x A_g [0.85 x 3 (1- .02) + .02 x 40]

A_g = 207.83 in

Size of column = 10″ x 21″

Provide, Ag = 10″ x 21″= 210 in

So, ok.

c) Calculation of steel:-

P_u = [0.85 f′c (A_g – A_st) + A_st fy]

Or, 356.54 = .80 x .65 x [.85 x 3 x (210- A_st) + A_st x 40]

A_st= 4.00 in

Use 10 # 6 bar straight.

Tie bar:-

Use # 3 bar as Tie

S = 16 x = 12″ c / c

S = 48 x = 18″ c / c

S = Minimum width of column = 10″ c / c

We use # 3 bar @ 10″ c / c

Check for lateral tie:-

S = = 4.75″ < 6″

So, Ok

No latered ties are required.

COLUMN (C-10):-

Assume column size = 10″ x 15″ = 150 sq. inch

a) Load Calculation:-

Load from F. B – A3, D3 = = 15.95 kip

Load from F. B – D3, D4 = = 9.29 kip

Load from F. B – D3, B3 = = 13.08 kip

Self weight of Column = 150 x x 9′ = 1.41 k

Factored load = 15.95 + 9.29 + 13.08 + 1.41 x1.2

= 40.00 kip

Total column load for 6- story = 6 x 40 = 240 kip

b) Check for size:-

Pu = 240 kip (Story -1)

P_u = A_g [ .85 x f′c (1- ρg) + ρ_gf_y]

Or, 240 = .80 x .65 x Ag [.85 x 3 (1- 0.02) + .02 x 40]

A_g = 139.90 in

Size of column = 10″ x 14″

Provided size = 10″ x 15″= 150 Sq. inch.

Ok.

c) Calculation of steel:-

P_u = [0.85 f′c (A_g – A_st) + A_st f_y]

Or, 240 = .80 x 0.65 [.85 x 3 (150- A_st) + A_stx 40]

A_st = 2.11 in

Use 4 # 7 bar st, Ap = 2.40in

Tie bar:

Use # 3 bar as Tie

S = 16 x = 14″ c / c

S = 48 x = 18″ c / c

S = Minimum width of column = 10″ c / c

We use # 3 bar @ 10″ c / c

Table:- 4.4. Details of sectional dimensions and reinforcement arrangement of all Columns (C – 1 ~ C – 10):

Columngroup	Numberof Column	ColumnSize	Columnload	Area of steel req. (sq.inch)	QuantityOf bars	Tie barsspacing
Columngroup	Numberof Column	ColumnSize	Pu (kip)	Ast	Main bars	Use # 3 bars
C – 1	02	10″ X 30″	516.34	6.10	14 # 6	10″ C/c
C – 2	02	10″ X 18″	308.96	3.60	8 # 6	10″ C/c
C – 3	02	10″ X 18″	289.77	2.52	6 # 6	10″ C/c
C – 4	02	10″ X 20″	342	3.95	10 # 6	10″ C/c
C – 5	02	10″ X 12″	189	1.53	6 # 5	10″ C/c
C – 6	02	10″ X 12″	152.5	0.34	4 # 5	10″ C/c
C – 7	02	10″ X 15″	246.42	2.27	4 # 7	10″ C/c
C – 8	02	10″ X 24″	407.82	4.59	8 # 7	10″ C/c
C – 9	02	10″ X 21″	356.54	4.00	10 # 6	10″ C/c
C- 10	02	10″ X 15″	240.00	2.11	4 # 7	10″ C/c

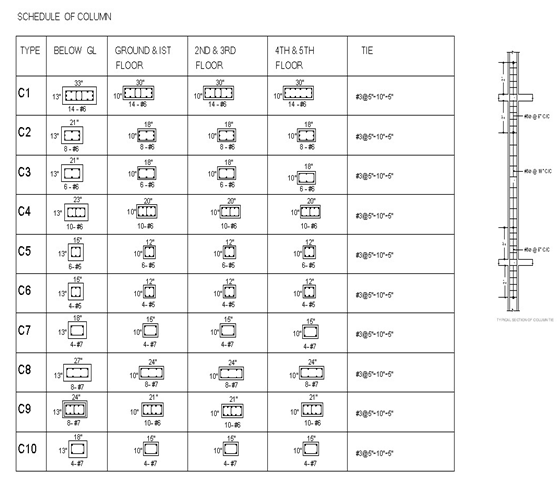
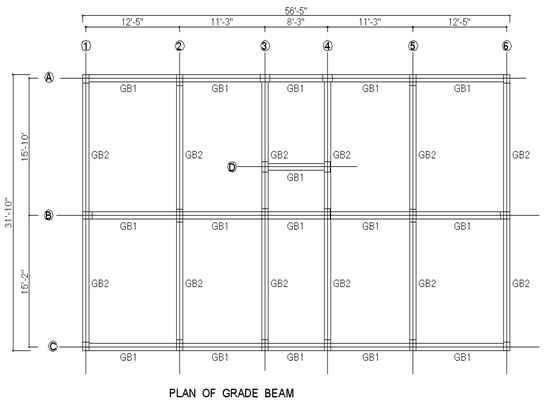
Figure Plan of Grade beam

Design of grade beams:

Design Data:–

Materials:-

F_y = 40 ksi

f′c = 3 ksi

w_c = 150 pcf

w_brick = 120 pcf

Thickness of wall on GB = 5″

Clear cover = 3″

Grade Beam GB-1:

Size = 10″ x 15″

Effective depth (d) = 15-3 = 12 inch

a) Load Calculation:-

Self weight of beam GB-1 = x 150 = 156.25 Ib/ft

All main wall weight = ( 10- )x120 = 430.00 Ib/ft

Total Dead load = 156.25 + 450 = 606.25 Ib/ft

Factored load GB-1 = 1.2 DL = 1.2 x 606.25

= 727.5 Ib/ft

= 0.73 k/ft

b) Moment Calculation and ‘d’ check:-

(-) Ve moment at Ext. support = = 7.04 k-ft

(+) Ve moment at Mid span = = 8.04 k-ft

(-) Ve moment at Int. support = = 11.26 k-ft

M_u= 11.26 k-ft

_b = 0.85ß₁ x x

= 0.85 x 0.85 x x

= 0.037

_max = 0.75 _b = 0.75 x 0.037 = 0.028

M_u = bd f_y (1-0.59 x

Or 11.26 x 12 = 0.9 x 0.028×12 x d x 40 x (1-0.59 x .028 x

= 3.78″ < 12″

So, ok

c) Reinforcement Calculation:

Mid span steel:-

= = 62.03 < 200 psi

_min = = = 0.005

_min= = = 0.0041

A_s = bd = 0.005x 12 x 12 = 0.72 inch

Use 2 # 6 hanger bar.

Steel for Int. Support:-

= = 86.88 psi < 200 psi

_min = 0.005

_min = 0.0041

A_s = bd = 0.005x 12 x 12 = 0.72 inch

Use 2 # 6 hanger bar.

Steel for Ext. Support:-

= = 54.32 psi < 200 psi

A_s = bd = 0.005x 12 x 12 = 0.72 inch

Use 2 # 6 hanger bar.

d) Stirrup design:-

V_v = 1.15 x = 4.86 K

V_u = 4.86 – ( ) x.73 = 4.13 k

V_c = 2 b_w d = 2 x 10 x 12 = 13.14 k

Shear strength of concrete,

V_c = .75 x 13.14 = 9.86 K

Since, V_c > V_d; no shear reinforcement is required.

Use # 3 bar @ = = 6″ c / c throughout beam length.

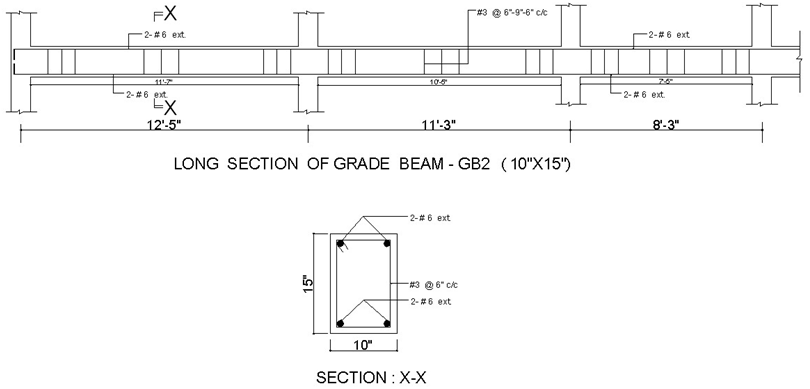
Grade Beam GB-2:

Size = 12″ x 15″

Effective depth, (d) = 15-3 = 12 inch

a) Load Calculation:-

Self weight of beam GB-2 = x 150 = 156.25 Ib/ft

All main wall weight = ( 10- ) x120 = 450.00 Ib/ft

Total Dead load = 156.25 + 450.00 = 606.25 Ib/ft

Factored load GB-2 = 1.2 DL = 1.2 x 606.25

= 727.5 Ib/ft

= 0.73 k/ft

b) Moment Calculation and d check:-

(-) Ve moment at Ext. support = = 11.43 k-ft

(+) Ve moment at Mid span = = 13.07k/ft

(-) Ve moment at Int. support = = 18.79 k-ft

Now, M_u = 18.79 k-ft

_b = .85x ₁ x x

= 0.85 x 0.85 x x

= 0.037

_max = 0.75 x 0.037 = 0.028

M_u = bd fy (1-0.59 x

Or 18.79 x 12 = 0.9 x .028×12 x d x 40 x (1-0.59 x .028 x

= 4.88″ < 12″

So, ok

c) Reinforcement Calculation:

Mid span steel:-

= = 100.84 < 200 psi

_min = = = 0.005

_min = = = 0.0041

A_s = bd = .005x 12 x 12 = 0.72

Use 2 # 6 bar hanger

Steel for Int. Support:-

= = 144.98 psi < 200 psi

_min = 0.005

A_s = 0.005x 12 x 12 = 0.72 inch

Use 2 # 6 bar hanger.

Steel for Ext. Support:-

= = 88.19 psi < 200 psi

_min = 0.005

A_s = 0.005x 12 x 12 = 0.72 inch

Use 2 # 6 bar hanger .

d) Stirrup design:-

V_u = 1.15 x = 6.29 K

V_u = 6.29 – ( ) x .73 = 5.57 k

Vc = 2 b_wd = 2 x 10 x 12 = 13.14 k

Shear strength of concrete,

V_c = 0.75 x 13.14 = 9.86 K

Since, V_c > V_d; no shear reinforcement is required.

Use # 3 bar @ = = 6″ c / c throughout beam length.

v\:* {behavior:url(#default#VML);}
o\:* {behavior:url(#default#VML);}
w\:* {behavior:url(#default#VML);}
.shape {behavior:url(#default#VML);}

Normal
0

false
false
false

EN-US
X-NONE
X-NONE

/* Style Definitions */
table.MsoNormalTable
{mso-style-name:”Table Normal”;
mso-tstyle-rowband-size:0;
mso-tstyle-colband-size:0;
mso-style-noshow:yes;
mso-style-priority:99;
mso-style-qformat:yes;
mso-style-parent:””;
mso-padding-alt:0in 5.4pt 0in 5.4pt;
mso-para-margin:0in;
mso-para-margin-bottom:.0001pt;
mso-pagination:widow-orphan;
font-size:10.0pt;
font-family:”Calibri”,”sans-serif”;}
table.MsoTableGrid
{mso-style-name:”Table Grid”;
mso-tstyle-rowband-size:0;
mso-tstyle-colband-size:0;
mso-style-priority:59;
mso-style-unhide:no;
border:solid windowtext 1.0pt;
mso-border-alt:solid windowtext .5pt;
mso-padding-alt:0in 5.4pt 0in 5.4pt;
mso-border-insideh:.5pt solid windowtext;
mso-border-insidev:.5pt solid windowtext;
mso-para-margin:0in;
mso-para-margin-bottom:.0001pt;
mso-pagination:widow-orphan;
font-size:10.0pt;
font-family:”Times New Roman”,”serif”;
mso-fareast-font-family:”Times New Roman”;}

Figure Reinfrocement Grade Beam ( GB-2 )

Table : Details of sectional dimensions and reinforcement arrangement of all grade beams ( GB- 1 GB – 2).

Grade Beam Group	Grade Beam Size	Moment (Kip-ft)			Area of steel req. (Sq. inch)			Quantity of bars		Stirrups (Spacing” C/c)
		At Ext. M-Ve	At Mid M + Ve	At Int. M-Ve	At Ext. As-Ve	At mid As+Ve	At Int. M-Ve	Main bars	Extra Top/bottom
GB – 1	10″ x 15″	7.04	8.04	11.26	0.72	0.72	0.72	At Ext, Suppt: 2 # 6	–	@ 6″ C/c
								At Mid, Span: 2 # 6	–	@ 6″ C/c
								At Int, Suppt: 2 # 6	–	@ 6″ C/c
GB – 2	10″ x 15″	11.43	13.07	18.79	0.72	0.72	0.72	At Ext, Suppt: 2 # 6	–	@ 6″ C/c
								At Mid, Span: 2 # 6	–	@ 6″ C/c
								At Int, Suppt: 2 # 6	–	@ 6″ C/c

Some are parts:

Analysis and Design of Building Components (Part 1)

Analysis and Design of Building Components (Part 2)

Analysis and Design of Building Components (Part 3)

Analysis and Design of Building Components (Part 3)

you might also like

Single Phase Transformer

Generation of Electricity by Wind Energy

Construction Sites

Development of Improved I-V Characteristics Models

Network Simulator Modifying NS2 for Evaluating QoS Parameters