INTRODUCTION
General:
When a slab is supported directly on columns, without beams and girders, it is called a flat plate slab. Although thicker and more heavily reinforced than slabs in beam and girder construction, flat plate slabs are advantageous because they offer no obstruction to passage of light (as beam construction does); savings in story height and in the simpler form work involved; less danger of collapse due to overload; and better fire protection with a sprinkler system because the spray is not obstructed by beams.
Objective of the Study:
The objectives of the study were:
To analyze and design a six-storied beam supported building system.
To analyze and design the same building for flat plate slab system.
To compare the concrete and steel requirement of the two types of building.
Methodology
i. Analysis Phase
Requires extensive FEM analysis
Correction of analysis results for BNBC since in the software we used UBC* 94 code
Development of shear force & moment envelope to determining the critical sections & values of critical shear and moment for design
ii. Design & Estimation Phase
Slab, Beam & Column Design & Estimation through manual calculation
Organization of the Thesis Works:
The thesis comprises of the following five chapters:
Chapter- 1: Includes a brief introduction, objectives of the study and organizations of the
thesis paper.
Chapter- 2: Includes compilation of the relevant literature that has been reviewed for the
study.
Chapter- 3: Includes a detailed description of the analysis.
Chapter- 4: Includes the comparison of the results of analysis.
Chapter- 5: Includes conclusions and recommendations for further study.
CHAPTER II LITERATURE REVIEW
Introduction
This chapter elaborated a detailed literature review that was required for the through understanding and proper conducting of this work.
Wind Load
The minimum deign wind load on buildings and components shall be determined based on the velocity of the wind, the shape and size of the building and the terrain exposure condition of the site. The design wind load shall include the effects of the sustained wind velocity component and the fluctuating component due to gusts. For slender buildings, the design wind load shall also include additional loadings effects due to wind induced vibrations of the building.
Terrain exposure
A terrain exposure category that adequately reflects the surface roughness characteristics of the ground shall be determined for the building site, taking into account the variations in ground roughness arising from existing natural topography, vegetation and man made constructions. The exposure category is divided into three types-
- Exposure A: Urban and sub-urban areas, industrial areas, wooded areas, hilly or other terrain covering at least 20 percent of the area with obstructions of 6 m or more in height and extending from the site at least 500 m or10 times the height of the structure whichever is greater.
- Exposure B: Open terrain with scattered obstructions having heights generally less Than 10 m extending 800 m or more from the site in any full quadrant. This category includes air fields, open park lands, sparsely built-up outskirts of towns, flat open country and grasslands.
- 3. Exposure C: Flat and unobstructed open terrain, coastal areas and riversides facing Large bodies of water, over 1.5 km or more in width, it extends inland from the
shoreline 400m or 10times the height of structure, whichever is greater.
Wind pressure on building:
Wind is one of the significant forces of nature that must be considered in the design of buildings. Structural load applied by high winds is readily appreciated, even if the method of determining them is not so easily understood. Other effects that can be caused even by moderate breezes are commonly overlooked, however, because very often there is no obvious link between wind and the behavior of a building.
Rain leakage around flashings and through joints in curtain walls may be due to a pressure gradient across the wall and the functioning of ventilating and heating systems may be affected by pressure distributions where ducts and openings are located.
Thus it is only the structural engineer who must consider wind action but the architect and mechanical engineer as well. The latter are often concerned with the maximum pressures that can reasonably by expected to occur during the useful life of the structure.
Conversion from wind speed to wind pressure:
Wind pressures exerted on a structure depend on the speed of the wind as well as the interaction between the airflow and the structure. The wind speed to be used in computing the design pressure depends on the particular component of the building being designed. For structural purposes the maximum value is required and will vary with the geographical location. Meteorological records of wind speed are analyzed to yield the most probable maximum that will be equaled or exceeded, on the average, once during a given period of a time comparable to the life of a structure.
Sustained Wind pressure
Qz = Cc * Ci* C z* Vb2
Where, Qz = Sustained wind pressure at height z, KN / m2
Ci = Structural importance coefficient
Cc = Velocity – to – pressure conversion
Cz = Combined height & exposure coefficient
Vb = basic wind speed in Km / hr
Design wind pressure:
Pz = Cg * Cp* Qz
Where,
Pz = design wind pressure at height z, KN / M2
Cg = gust coefficient
Cp = pressure coefficient for structures or components
Qz = sustained wind pressure
Pressure coefficients
Pressure coefficients used in practice have usually been obtained experimentally by testing models of different types of structures in wind tunnels. Commonly used coefficients refer to the average pressure or suction over a surface. Tangential forces are considered insignificant, so that the forces referred to act at right angles to the surfaces in question.
Variables affecting pressure distributions
Building shape:
Pressure on certain parts of a structure is rather sensitive to changes in the shape of the building. The suctions on the windward roof slope, for instance, very considerably with the slope of the roof, the ratio of height to width, and the ratio of width to length of the building. Suctions on the leeward wall, on the other hand, are not greatly affected by such variables.
Sometimes shape details have an unexpectedly large effect on the wind pressure distribution. Parapet walls, large chimneys, silos and spires may have considerable influence and often the only way to assess such effects is to test a scale model in a wind tunnel.
Openings:
The size and location of opening such as windows and doors determine the internal pressure that must be considered in the calculation of net forces of walls and roofs. Internal pressure tend to take on the values appropriate to the exterior of the wall in which in which the opening predominate. If they are small and uniformly distributed, values of ± 2 are recommended, the more unfavorable of the two to be considered in each case.
Wind direction:
The orientation of a building to the wind has a market effect on pressure distribution, particularly on suction maxima, which occur over a small area near the leading edges of roofs.
Increase of wind speed with height:
Since the wind speed and consequently the velocity pressure increase with height above the ground, a height factor is applied to the basic pressure in the design of building.
Shielding:
Other buildings, trees and similar large objects in the immediate vicinity have a bearing on pressure distribution. The shielding provided is usually difficult to estimate and model tests provide the most convenient means of determining design values. The assignment of reduction for shielding is completed by the fact that conditions could change during the life of the structure. Shielding does not always has a beneficial effect, and in some cases suction coefficients should be increased because of the proximity of a neighboring building.
Wind pressures on various part of building
Roofs:
The roof is usually the critical area in the wind design of low building, particularly residential structures. Where it is made up of light- weight components particular attention must be paid to anchorage details because of the suction condition prevailing over most, if not all, of it. A good example of such precautions is the time –honored custom of weighting roofs in alpine areas with large stones.
Critical angle, windward slope:
For every sloped roof there is a certain slope angle at which the suction coefficients over the windward slope reaches a numerical maximum.
Steep roofs:
As the roof slope increases beyond the critical angle the average pressure coefficient decreases numerically to zero; it the increases in a positive direction, indicating pressure, to maximum of + 0.8 or so for a slope angle of 90 degrees.
Leeward slope:
The effect of slope and building dimension ratios are much less pronounced of suctions on the leeward slope and for general purposes could probably be disregarded.
Local suctions:
Local suctions are more serious for wind at an angle (usually about 45 degree) to the side of the building.
Walls:
For tall, slender structures the design of the walls and the frame, with regard to overturning moment, are likely to be critical. The trend toward high-rise buildings and
curtain wall construction may lead to greater problems in limiting sway and specifying the strength of fastenings for the wall panels.
Earthquake Load
Minimum design earthquake forces for buildings, structures or components of buildings or structures, can be calculated either by the Equivalent static force method of by the Dynamic response method. We will calculate earthquake load by equivalent static force method.
Seismic zoning map
The seismic zoning map of Bangladesh is provided by BNBC. Based on the severity of the probable intensity of seismic ground motion and damages, Bangladesh has been divided into three seismic zones.
These are –
- Zone-1
- Zone-2
- Zone-3
Selection of lateral force method
Seismic lateral forces on primary framing systems shall be determined by using either the equivalent static force method of the dynamic response method with the restriction given bellow-
a) The equivalent static force method
- All structures, regular or irregular, in seismic zone 1 and in structure importance
- Regular structures less than 75 m in height with lateral force resistance provided by structural systems listed in BNBC except case 4 below.
- Irregular structures not mire than 20 m in height.
- A tower like building or structure having a flexible upper portion supported on a rigid lower portion where:
- Both portion of the structure considered separately can be classified as regular structures,
- The average story stiffness of the lower portion is at least tin times the average storey stiffness of the upper portion.
- The period of the entire structure is not greater than 1.1 times the period of the upper portion considered as a separate structure fixed at he base.
b) The Dynamic response method shall be used for structures of the following types-
- Structures 75 m or more in height except as permitted by case a (1).
- Structures having stiffness, weight or geometric vertical irregularity of type 1, 2& 3 is defined in the BNBC table or structures not described.
- Structure over 20 m in height in seismic zone 3 not having the same structural
system throughout their height except as permitted by BNBC.
- Structures, regular or irregular, located on soil profile type S4 as described, which have a period greater than .1 second. The analysis shall include the effect of the soil at the site.
Seismic dead load
Seismic dead load, W, is the total dead load of a building or a structure, including permanent partitions and applicable portions of other loads listed below:
1) In storage and warehouse occupancies, a minimum of 25 percent of the floor live
load shall be applicable.
2) Where an allowance for partition load is include in the floor design in accordance
with BNBC all such loads but not less than .6 KN / m2 shall be applicable.
3) Total weight of permanent equipment shall be included
Equivalent static force method
Design base shear:
V = (ZIC / R) * W
Where,
Z = Seismic zone coefficient
I = Structure importance coefficient
R = Response modification coefficient for structural systems
W = Total seismic dead load
C = Numeric coefficient given by the relation-
C = (1.25 S) / T2/3
Where,
S = Site coefficient for soil characteristics
T = Fundamental period of vibration in seconds
Where, T = Ct (hn) 3/4
Ct = 0.083 for steel moment resisting frames
= 0.073 for reinforced concrete moment resisting frames and
Eccentric braced steel frames.
= 0.049 for all other structural systems.
hn = Height in meters above the base to level n
Vertical distribution of lateral force
In the absence of a more rigorous procedure, the total lateral force, which is the base shear V, shall be distributed along the height of the structure in accordance with the following equation:
V = Ft + ∑ Fi
Where,
Fi = Lateral force applied at storey level I
Ft = Concentrated lateral force considered at the top of the building in
Addition to the force Fn
Where,
Ft = 0.070 TV ≤ 0.25V When T > 0.70 second
Ft = 0.0 When T ≤ 0.70 second
The remaining portion of the base shear (V-Ft) shall be distributed over the height of the building, including level – n, according to the relation:
Fi = (V- Ft) * W l * hi) / ∑Wi * hi
Direct Design Method
Assumption
Moments in two way slabs can be found using the semi empirical direct design method, subjected to the following restrictions:
- There must be a minimum of three continuous spans in etch direction.
- The panels must be rectangular, with the ratio of the longer to the shorter spans
within a panel not greater than 2.
- The successive span lengths in each direction must not differ by more than one-third
of the longer span.
- Columns may be offset a maximum of 10 percent of the span in the direction of the
offset from either axis between center lines of successive columns.
5. Loads must be due to gravity only and the live load must not exceed two times the
dead load.
6. If beams are used on the column lines, the relative stiffness of the beams in the two perpendicular directions, given by the ratio: α1l2² / α2l12, must be between 0.2 and 5.0
Static moment
For purpose of calculating the total static moment Mo in a panel, the clear span ln in the direction of moments is used. The clear span is defined to extend from face to face of the columns, capitals, brackets, or walls but is not to be less than 0.65 l1. The total factored moment in a span, for a strip bounded laterally by the centerline of the panel on each side of the centerline of supports is-
Mo = wu l2 ln² / 8
Reciprocal Method
A simple, approximate design method developed by Bresler has been satisfactorily verified by comparison with results of extensive tests and accurate calculation. The column interaction surface can alternatively be plotted as a function of the axial load Pn and eccentricities ex = Mny / Pn and ey = Mnx / Pn, The surface S1 of fig-a can be transformed into an equivalent failure surface S2, as shown in fig – b, where ex and ey are plotted against 1 / Pn rather than Pn. Thus, ex = ey = 0 corresponds to the inverse of the capacity of the column if it were concentrically loaded, Po and this is plotted as point C. For ey = 0 and any given value of ex, there is a load Pnyo that would result in failure. The reciprocal of this load is plotted as point A. Similarly , for ex = 0 and any given value of ey, there is a certain load Pnxo that would cause failure, the reciprocal of which is point B. The values of Pnxo and Pnyo are easily established, for known eccentricities of loading applied to a given column, using the methods already established for uniaxial bending or using design charts for uniaxial bending. An oblique plane S2 is defined by the three points A, B, C. This plane is used as an approximation of the actual failure surface.
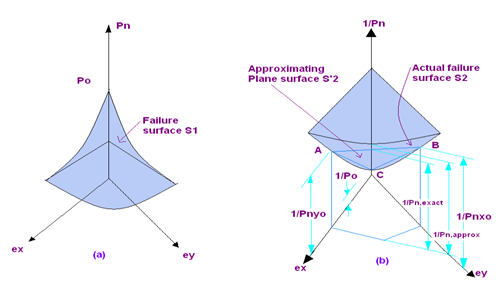
Figure 2.1: Interaction surfaces for the reciprocal load method.
The vertical ordinate 1 / Pn, exact to the true failure surface will always be conservatively estimated by the distance 1 / Pn, approx to the oblique plane ABC because of the concave upward eggshell shape of the true failure surface. In other words,
1 / Pn, approx is always greater than 1 / Pn, exact which means that Pn, approx is always less than Pn, exact. Breslers reciprocal load equation derives from the geometry of the approximating plane, it can be shown that-
1 / Pn = 1 / Pnxo + 1 / Pnyo + 1 / Po
Where, Pnxo = nominal load when only eccentrically ey is present (ex = 0)
Pnyo = nominal load when only eccentrically ex is present (ey = 0)
Po = nominal load for concentrically loaded column.
The above equation has been found to be acceptably accurate for design purposes provided ρn ≥ 0.10ρo. It is not reliable where biaxial bending is prevalent and accompanied by an axial force smaller than ρo / 10.
Analysis and Design Basis:
This thesis is prepared properly based on Bangladesh National Building Code. Every part of this thesis is properly maintained the recommendation of this code. Here some features are described below-
Bangladesh country paper for WCDR 7
Does your country have building codes of practice and standards in place?
Which takes into account seismic risk?
The National Building Code was formulated and published in 1993. Bangladesh does not have any separate code for the design or construction of earthquake resistant structure. However, a new seismic zoning map and detailed seismic design provisions were incorporated into the National Building Code in 1993 that replaces the code prepared in 1979. The Bangladesh Earthquake Society has recently published a Bengali translation of the Guidelines for Earthquake Resistant Non-Engineered Construction, written by the International Association of Earthquake Engineering 3. The enforcement of the standards presented in the National Building Code requires close monitoring by concerned agencies. The shortage of trained staff to monitor new construction impedes the effectiveness of the building standards.
Analysis Software:
There is much finite element software for analyzing structure. ETABS is one of them. Every analysis is this thesis is done by using ETABS 8 package. In the following paragraph we will discuss some of its features-
Introduction
ETABS is a sophisticated, yet easy to use, special purpose analysis and design program developed specifically for building systems. ETABS version 8 features an intuitive and powerful graphical interface coupled with unmatched modeling, analytical and design procedures, all integrated using a common database. Although quick and easy for simple structures, ETABS can also handle the largest and most complex building models, including a wide range of nonlinear behaviors, making it the tool of choice for structural engineers in the building industry.
History and advantages of ETABS
Dating back more than 30 years to the original development of TABS, the predecessor of ETABS, it was clearly recognized that buildings constituted a very special class of structures. Early releases of ETABS pro-vided input, output and numerical solution techniques that took into consideration the characteristics unique to building type structures, providing a tool that offered significant savings in time and increased accuracy over general purpose programs. As computers and computer interfaces evolved, ETABS added computationally complex analytical options such as dynamic nonlinear behavior, and powerful CAD-like drawing tools in a graphical and object-based interface. Although ETABS Version 8 looks radically different from its predecessors of 30 years ago, its mission remains the same: to provide the profession with the most efficient and comprehensive software for the analysis and design of buildings. To that end, the current release follows the same philosophical approach put forward by the original programs, namely: Most buildings are of straightforward geometry with horizontal beams and vertical columns. Although any building configuration is possible with ETABS, in most cases, a simple grid system defined by horizontal floors and vertical column lines can establish building geometry with minimal effort. Many of the floor levels in buildings are similar. This commonality can be used numerically to reduce computational effort. The input and output conventions used correspond to common building technology. With ETABS, the models are defined logically floor-by-floor, column, bay-by-bay and wall-by-wall and not as a stream of non-descript nodes and elements as in general purpose programs. Thus the structural definition is simple, concise and meaningful. In most buildings, the dimensions of the members are large in relation to the bay widths and story height.
Those dimensions have a significant effect on the stiffness of the frame ETABS corrects for such effects in the formulation of the member stiff-ness, unlike most general-purpose programs that work on center-line-to-center-line dimensions. The results produced by the programs should be in a form directly usable by the engineer. General purpose computer programs produce result in a general form that may need additional processing before they are useable in structural design.
An integrated approach
ETABS is a completely integrated system. Embedded beneath the simple, intuitive user interface are very powerful numerical methods, design procedures and international design codes, all working from a single comprehensive database. This integration means that you create only one model of the floor systems and the vertical and lateral framing system to analyze and design the entire building. Everything you need is integrated into one versatile analysis and design package with one Windows-based graphical user interface. No external modules are maintained, and no data is transferred between programs or modules. The effects on one part of the structure from changes in another part are instantaneous and automatic. The integrated modules include:
- Drafting module for model generation.
- Seismic and wind load generation module.
- Gravity load distribution module for the distribution of vertical loads to columns and beams when plate bending floor elements are not provided as a part of the floor system.
- Finite element-based linear static and dynamic analysis module.
- Finite element-based nonlinear static and dynamic analysis module.
- Output display and report generation module.
- Steel frame design module (column, beam and brace).
- Concrete frame design module (column and beam).
- Composite beam design module
- Steel joist design module
- Shear wall design module.
Modeling features
The ETABS building is idealized as an assemblage of area, line and point object. Those objects are used to represent wall, floor, column, beam, brace and link/spring physical members. The basic frame geometry is defined with reference to a simple three dimensional grid system. With relatively simple modeling techniques, very complex farming situations may be considered. The buildings may be unsymmetrical and non-rectangular in plan. Torsional behavior of the floors and inter story compatibility of the floors are accurately reflected in the result. The solution enforces complete three dimensional displacement compatibility, making it possible to capture tubular effects associated with the behavior of tall structures having relatively closely spaced columns. Semi-rigid floor diaphragms may be modeled to capture the effects of in plane floor deformations. Floor objects may span between adjacent levels to create sloped floors (ramps), which can be useful for modeling parking garage structures. Modeling of partial diaphragms, such as in mezzanines, setbacks, atriums and floor openings, is possible without the use of artificial (“dummy”) floors and column lines. It is also possible to model situations with multiple independent diaphragms at each level, allowing the modeling of buildings consisting of several towers rising from a common base. The column, beam and brace elements may be non-prismatic, and they may have partial fixity at their end connections. They also may have uniform, partial uniform and trapezoidal load patterns, and they may have temperature loads. The effects of the finite dimensions of the beams and columns on the stiffness of a frame system are included using end offsets that can be automatically calculated. The floors and walls can be modeled as membrane elements with in-plane stiffness only, plate bending elements with out-of-plane stiffness only or full shell-type elements, which combine both in-plane and out-of-plane stiffness. Floor and wall objects may have uniform load patterns in-plane or out-of-plane, and they may have temperature loads. The column, beam, brace, floor and wall objects are all compatible with one another.
Analysis features
Static analysis for user specified vertical and lateral floor or story loads are possible. If floor elements with plate bending capability are modeled, vertical uniform loads on the floor are transferred to the beams and columns through bending of the floor elements. Otherwise, vertical uniform loads on the floor are automatically converted to span loads on adjoining beams, or point loads on adjacent columns, thereby automating the tedious task of transferring floor tributary loads to the floor beams without explicit modeling of the secondary framing. The program can automatically generate lateral wind and seismic load patterns to meet the requirements of various building codes. Three-dimensional mode shapes and frequencies, modal participation factors, direction factors and participating mass percentage are evaluated using eigenvector. P-Delta effects may be included with static or dynamic analysis. Response spectrum analysis, linear time history analysis, nonlinear time history analysis, and static nonlinear (pushover) analysis are all possible. The static nonlinear capabilities also allow you to perform incremental construction analysis so that forces that arise as a result of the construction sequence are included. Result from the various static load conditions may be combined with each other or with the result from the dynamic response spectrum or time history analysis. Output may be viewed graphically, displayed in tabular output, sent to a printer, exported to a database file, or saved in an ASCII file. Types of output include reactions and member forces, mode shapes and participation factors, static and dynamic story displacements and story shears, inter story drifts and joint displacements, time history traces, and more.
Shell element internal forces
The shell element internal forces, like stresses, act throughout the element. They are present at every point on the mid surface of the shell element. ETABS reports values for the shell internal forces at the element nodes. It is important to note that the internal forces are reported as forces and moments per unit of in-plane length. The basic shell element forces and moments are identified as F11, F22, F12, M11, M22, M12, V13 and V23. You might expect that there would also be an F21 and M21, but F21 is always equal to F12 and M21 is always equal to M12, so it is not actually necessary to report F21and M21.
Conclusion
Materials problem is a great problem in our country especially the shortage of constriction raw materials in our country. This thesis is based on the previously discussed topics.
This thesis may result an effective solution of this problem.
ANALYTICAL STUDY
General:
The analysis is made by using ETABS finite element package. Analysis was made for two different types of building systems. One is beam column slab system and another is flat plate slab system. Total ten loads combination was considered for design of different elements of the building. The whole analysis and design was performed based on ACI and BNBC code.
The Building Geometry
The building geometries are as follows:
Option I:
All the floors have 16 columns. All the slabs of the structure are beam supported. Story height is 10 ft. column and beam size is different. The layout is shown in figure 3.2
Option II:
All the floors have 16 columns. All the slabs are directly supported on column (Flat Plate structure). Column size is different. The layout is shown in figure 3.1
The Loads Considered
Dead Load, D. L = 50 psf (for wall)
Floor Finish, F. F = 30 psf
Live Load, L. L = 40 psf
Load Combination
COMB 1 = 1.4 D.L
COMB 2 = 1.4 D.L + 1.7 L.L
COMB 3 = 0.75 (1.4 D.L + 1.7 L.L + 1.7 WLX)
COMB 4 = 0.75 (1.4 D.L + 1.7 L.L. – 1.7 WLX)
COMB 5 = 0.75 (1.4 D.L + 1.7 L.L + 1.7 WLY)
COMB 6 = 0.75 (1.4 D.L + 1.7 L.L – 1.7 WLY)
COMB 7 = 0.75 (1.4 D.L + 1.7 L.L + 1.87 ELX)
COMB 8 = 0.75 (1.4 D.L + 1.7 L.L – 1.87 ELX)
COMB 9 = 0.75 (1.4 D.L + 1.7 L.L + 1.87 ELY)
COMB 10 = 0.75 (1.4 D.L + 1.7 L.L – 1.87 ELY)
Legends:
D.L = Dead load
L.L = Live load
WLX = Wind load in X direction
WLY = Wind load in Y direction
EQX = Earthquake load in X direction
EQY = Earthquake load in Y direction
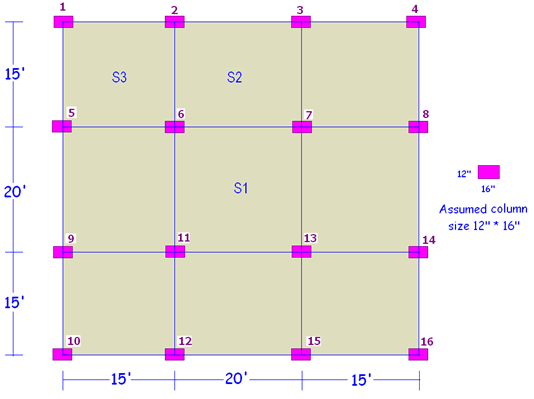
Figure 3.1: Option II (Typical floor plan of the flat plate structure).
Wind Load Calculation
This load is a function of the wind speed which in turn is depended on the location of the building, the exposure of the location, gusting effect, importance of the building and the geometry of the building. Wind load calculations were done by UBC – 94 codes by ETABS. The wind speed was adjusted to convert it to BNBC code. In this study, wind load was calculated by the diaphragms method.
Input data
Windward Coefficient, Cq = 1.4
Leeward Coefficient, Cq ≈ 0
Wind Speed, V = 210 km / hr (131.25 mph)
Exposure Type = B, Importance Factor = 1.0
Along X axis, Wind Direction Angle = 00
Along Y axis, Wind Direction Angle = 900
Velocity adjustment
The table below shows the reaction due to wind load in X and Y direction.
Table 3.1: ETABS output value for reaction forces:
|
Story |
Load |
Fx |
Fy |
|
BASE |
WLX |
– 7.56 |
– 0.27 |
|
BASE |
WLY |
0.27 |
– 8.11 |
|
BASE |
WLX |
– 10.27 |
– 0.20 |
|
BASE |
WLY |
0.20 |
– 8.03 |
|
BASE |
WLX |
– 10.68 |
– 0.20 |
|
BASE |
WLY |
– 0.20 |
– 8.03 |
|
BASE |
WLX |
– 8.11 |
– 0.27 |
|
BASE |
WLY |
– 0.27 |
– 8.11 |
|
BASE |
WLX |
– 8.03 |
– 0.20 |
|
BASE |
WLY |
– 0.20 |
– 10.68 |
|
BASE |
WLX |
– 10.63 |
– 0.15 |
Table 3.1: ETABS output value for reaction forces (continued…).
|
Story |
Load |
Fx |
Fy |
|
BASE |
WLY |
– 0.15 |
– 10.63 |
|
BASE |
WLX |
– 10.33 |
– 0.15 |
|
BASE |
WLY |
0.15 |
– 10.63 |
|
BASE |
WLX |
-7.64 |
– 0.20 |
|
BASE |
WLY |
0.20 |
– 10.68 |
|
BASE |
WLX |
– 7.64 |
0.20 |
|
BASE |
WLY |
0.20 |
– 10.27 |
|
BASE |
WLX |
– 7.56 |
0.27 |
|
BASE |
WLY |
0.27 |
– 7.56 |
|
BASE |
WLX |
– 10.33 |
0.15 |
|
BASE |
WLY |
0.15 |
– 10.33 |
|
BASE |
WLX |
– 10.27 |
0.20 |
|
BASE |
WLY |
0.20 |
-7.64 |
|
BASE |
WLX |
– 10.63 |
0.15 |
|
BASE |
WLY |
– 0.15 |
– 10.33 |
|
BASE |
WLX |
– 10.68 |
0.20 |
|
BASE |
WLY |
– 0.20 |
– 7.64 |
|
BASE |
WLX |
– 8.03 |
0.20 |
|
BASE |
WLY |
– 0.20 |
– 10.27 |
|
BASE |
WLX |
– 8.11 |
0.27 |
|
BASE |
WLY |
– 0.27 |
-7.56 |
|
Summation |
WLX |
– 146.48 |
0 |
|
Summation |
WLY |
0 |
– 146.48 |
From Hand Calculation:
Table 3.2: Wind force X / Y direction.
| height | Vb2 | qz | Pz | Pz | A | F | ||||||
| H(m) | Cc | Ci | cz | 210 | (KN/m2) | Cg | Cp | (KN/m2) | (psf) | (sft) | (Kips) | |
| F-1 | 3.05 | 4.7E-5 | 1 | 0.37 | 44100 | 0.76 | 1.38 | 1.4 | 1.48 | 30.92 | 250 | 7.73 |
| F-2 | 6.1 | 4.7E-5 | 1 | 0.41 | 44100 | 0.86 | 1.38 | 1.4 | 1.68 | 35.08 | 500 | 17.54 |
| F-3 | 9.15 | 4.7E-5 | 1 | 0.50 | 44100 | 1.03 | 1.38 | 1.4 | 2.01 | 41.97 | 500 | 20.99 |
| F-4 | 12.2 | 4.7E-5 | 1 | 0.57 | 44100 | 1.17 | 1.38 | 1.4 | 2.28 | 47.77 | 500 | 23.89 |
| F-5 | 15.24 | 4.7E-5 | 1 | 0.63 | 44100 | 1.30 | 1.38 | 1.4 | 2.52 | 52.74 | 500 | 26.37 |
| F-6 | 18.3 | 4.7E-5 | 1 | 0.68 | 44100 | 1.41 | 1.38 | 1.4 | 2.74 | 57.29 | 500 | 28.65 |
| Summation of F | 125.16 | |||||||||||
So the adjusted wind speed in both direction,
V = 131.25 * √ (125.16 / 146.48) = 121.32 mph
Table 3.3: The table below shows the reaction due to wind load in X and Y direction after correction of wind speed.
|
Story |
Load |
Fx |
Fy |
|
BASE |
WLX |
– 6.44 |
– 0.25 |
|
BASE |
WLY |
0.25 |
– 6.94 |
|
BASE |
WLX |
– 8.75 |
– 0.14 |
|
BASE |
WLY |
0.2 |
– 6.83 |
|
BASE |
WLX |
– 9.15 |
– 0.14 |
Table 3.3: The table below shows the reaction due to wind load in X and Y direction after correction of wind speed (continued…).
Design of beam supported slab
Design code: ACI.
Design method: USD
Design procedure: Direct design method
Slab system: Beam supported slab.
Material properties: fy = 60 ksi; fc’= 3.5 ksi
Unit wt. of concrete, wc = 150 psf
Unit wt. of brick, wb = 120 psf.
Loads: Live load = 40 psf,
Floor finish = 30 psf
Partition wall load = 50 psf
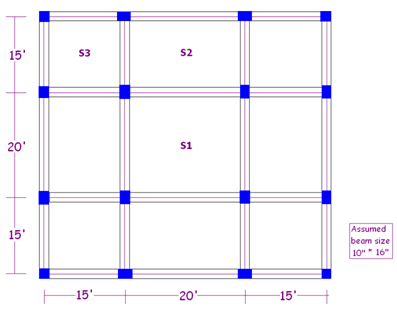
Figure 3.2: Option I (Typical floor plan of the beam supported structure)
Calculation of slab thickness:
Let, slab thickness h = 6 inch.
Beam size = 10 in * 16 in.
Now, 4hf = 24 in, hw = 10 in.
For the edge beam: I = 10 * 1.50 * 16³ * 1 / 12 = 5120 in4
For the interior beam: I = 10 * 2 * 16³ * 1 / 12 = 6827 in4
For the slab strips:
For the 7.92 ft edge width: I = 7.92 * 12 * 6³ * 1/12 = 1711 in4
For the 17.5 ft width: I = 17.50 * 12 * 6³ * 1 / 12 = 3780 in4
Thus for the edge beam α1 = 5120 / 1711 = 2.99
For the interior beam α2 = 6827 / 3780 = 1.81
Average value, αm = 2.40
For 15’*15’ slab panel: β = 1
For 15’*20’ slab panel: β = (20 – 10 / 12) / (15 – 10 / 12) = 19.17 / 14.17 = 1.35
For 20’*20’ slab panel: β = 1
Now, slab thickness h = [12 * 19.17{0.8+ (60000 / 200000)}] / [36 + 9 * 1] = 5.62 in.
The 3.50 in limitation clearly does not control in this case. 6 in. depth is ok.
Factored load, W = 285 psf.
For 15 ft * 15 ft panel:
Slab – beam strip centered on the Interior column line.
Mo = 0.285 * 17.50 *14.17² * 1 / 8 = 137 ft – kip.
Interior negative moment: 137 * 0.70 = 96 ft-kip
Positive moment: 137 * 0.57 = 78 ft – kip
Exterior negative moment: 137 * 0.16 = 22 ft – kip.
The torsional constant, C = {1 – (0.63 * 10 / 10)16 * 10³ / 3 + {1 – (0.63*6 / 10)10 * 6³ / 3
= 3681 in4
Now, l2 / l1 = 1; α1l2 / l1 = 1.81; βt = 3681 / (2*3780) = 0.50
Exterior negative moment 90%, positive moment 75%, Interior negative moment 75% is taken by column strip.
The table below is showing slab strip moment at different locations for 15’*15’ panel.
Table 3.5: Slab-beam strip centered on the Interior column line.
|
Slab-beam strip |
Column strip slab moment ft-kip |
Middle strip slab moment ft-kip |
|
Exterior negative moment |
3 |
2.2 |
|
Positive moment |
9 |
20 |
|
Interior negative moment |
11 |
24 |
Slab-beam strip at the edge of the building:-
Mo = 0.285 * 7.92 * 14.17² * 1 / 8 = 62 ft-kips
Interior negative: 62 * 0.70 = 43 ft-kips
Positive: 62 * 0.57 = 35 ft-kips
Exterior negative: 62 * 0.16 = 10 ft-kips
Now, l2 / l1= 1; α112 / l1 = 2.99 * 1 = 2.99; βt = 3681 / (2 * 1711) = 1.10
Positive moment 75%, Exterior negative moment 90%, Interior negative moment 75% is taken by column strip.
Table 3.6: Slab-beam strip at the edge of the building: (slab, 15′ * 15′).
|
Exterior slab-beam strip |
Column strip slab moment ft-kip |
Middle strip moment ft-kip |
|
Exterior negative moment |
1.50 |
1 |
|
Positive moment |
4 |
9 |
|
Interior negative moment |
5 |
11 |
For 15 ft * 20 ft Panel:
Slab-beam strip at the edge of the building.
Mo = 0.285 * 7.92 * 19.17² * 1 / 8 = 113 ft-kips.
Interior negative moment: 113 * 0.65 = 73.5 ft-kips
Positive moment: 113 * 0.35 = 39.5 ft-kip
Exterior negative moment: 137 * 0.16 = 22 ft-kip.
Now, l2 / l1 = 15 / 20 = 0.75; α1l2 / l1 = 2.99 * 0.75 = 2.24
Negative moment 83%, positive moment 83%, is taken by column strip.
Table 3.7: Slab-beam strips at the edge of the building (slab 15 ft * 20 ft).
|
Exterior Slab-beam strip (20 ft span) |
Column strip slab moment ft-kips |
Middle strip slab moment ft-kips |
|
Negative moment |
9 |
12.50 |
|
Positive moment |
5 |
7 |
Slab-beam strip centered on the Interior column line
Mo = 0.285 * 17.50 * 19.17² * 1 / 8 = 250 ft – kips
Negative: 250 * 0.65 = 163 ft – kips
Positive: 250 * 0.35 = 88 ft – kips
Now, l2 / l1= 15 / 20 = 0.75; α1l2 / l1 = 0.75 * 1.81 = 1.40;
Positive moment 83% and negative moment 83% is taken by column strip.
Table 3.8: Slab-beam strips centered on the Interior column line.
|
Interior slab-beam strip (20 ft span) |
Column strip slab moment ft-kips |
Middle strip moment ft-kips |
|
Negative moment |
20 |
28 |
|
Positive moment |
11 |
15 |
For 15 ft * 15 ft panel:
The table below is showing reinforcement requirement for different strip such as column strip, middle strip for 15’*15’ slab.
Table 3.9: Design of slab reinforcement.
|
Description |
Location |
Mu ft-kip |
Strip width b inch |
Effective depth d inch |
Mu*12/ b |
Steel density ρ |
Required steel area As in² |
Nos of # 3 bar |
|
Exterior half column strip |
Exterior: negative positive Interior: negative |
1.5 4 5 |
50 50 50 |
5 5 5 |
0.36 0.96 1.2 |
0.0022 0.0022 0.0022 |
0.55 0.55 0.55 |
6 6 6 |
|
Middle strip |
Exterior: negative positive Interior: negative |
2.1 19 23 |
90 90 90 |
5 5 5 |
0.28 2.53 3.1 |
0.0022 0.0022 0.0024 |
0.99 0.99 1.08 |
9 9 10 |
|
Interior half column strip |
Exterior: negative positive Interior: negative |
1.5 4.5 5.5 |
45 45 45 |
5 5 5 |
0.4 1.2 1.5 |
0.0022 0.0022 0.0022 |
0.5 0.5 0.5 |
5 5 5 |
|
Exterior half column strip |
Exterior: negative positive Interior: negative |
1.5 4 5 |
50 50 50 |
4.5 4.5 4.5 |
0.36 0.96 1.2 |
0.0024 0.0024 0.0024 |
0.54 0.54 0.54 |
6 6 6 |
Table 3.9: Design of slab reinforcement (continued…).
The table below is showing reinforcement requirement for different strip such as column strip, middle strip for 15 ft * 20 ft slab.
Table 3.10: Design of slab reinforcement.
|
Description |
Location |
Mu ft-kip |
Strip width b inch |
Effective depth of slab d inch |
Mu*12/b |
Steel density ρ |
Required steel area As in² |
Nos of # 3 bar |
|
15’span two half column strip |
Exterior: negative positive Interior: negative |
3 9 11 |
75 75 75 |
5 5 5 |
0.48 1.44 1.76 |
0.0022 0.0022 0.0022 |
0.83 0.83 0.83 |
8 8 8 |
|
Middle strip |
Exterior: negative positive Interior negative |
2.2 20 24 |
150 150 150 |
5 5
5 |
0.2 1.6 1.92 |
0.0022 0.0022 0.0022 |
1.65 1.65 1.65 |
15 15 15 |
Table 3.10: Design of slab reinforcement (continued…).
The table below is showing reinforcement requirement for different strip such as column strip, middle strip for 20 ft * 20 ft slab.
| Table 3.11: Design of slab reinforcement. | ||||||||
|
Description |
Location |
Mu ft-kip |
Strip width b inch |
Effective depth of slab d inch |
Mu*12/b Ft-kip/ft |
Steel density ρ |
Required steel area As in² |
Nos of # 3 bar |
|
Two half column strip |
Negative Positive |
20 11 |
120 120 |
5 5 |
2 1.1 |
0.0022 0.0022 |
1.32 1.32 |
12 12 |
|
Middle strip |
Negative Positive |
28 15 |
120 120 |
5 5 |
2.8 1.5 |
0.0022 0.0022 |
1.32 1.32 |
12 12 |
|
Two half column strip |
Negative Positive
|
20 11 |
120 120 |
4.5 4.5 |
2 1.1 |
0.0024 0.0024 |
1.3 1.3 |
12 12 |
|
Middle strip |
Negative Positive |
28 15 |
120 120
|
4.5 4.5
|
2.8 1.5 |
0.0026 0.0024
|
1.4 1.3 |
13 12
|
Minimum steel As min = 0.0018 * 12 * 6 = 0.13 in²
ρ min = 0.13 / (5 * 12) = 0.0022
Or ρ min = 0.13 / (4.5 * 12) = 0.0024
Maximum spacing = 2h = 2 * 6 = 12 in.
The figure below is showing the reinforcement arrangement in slab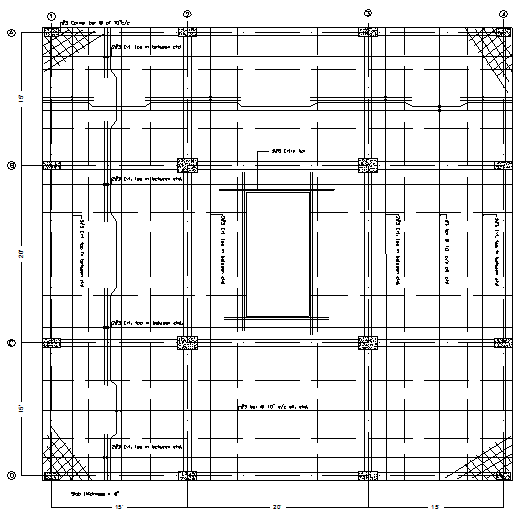
Figure 3.3: Reinforcement arrangement in slab (Beam Supported Structure).
Design of flat plate slab:
Thickness = ln / 30 = (20 * 12-16) / 30 = 7.5”
Dead Load
Self wt of slab: 7.50 * 12.5
Wall Load: 50 psf
Floor Finish: 30 psf
Total Dead Load: 173.75 psf
LL = 40 psf
Factored Load Wu = 1.4DL + 1.7LL = 311.25 psf
For Interior slab 20 ft * 20 ft:
Mo = (1 / 8) Wul2ln² = (1 / 8) * 311.25 * 20 * {20 – (16 / 12)}² = 271.2 kip-ft
Negative moment = 271.2 * 0.65 = 176.28 kip – ft
Positive moment = 271.2 * 0.35 = 94.92 kip – ft
Column strip negative: 0.75 * 176.28 = 132.21
Column strip positive: 0.60 * 94.92 = 56.95
Middle strip negative: 176.28 – 132.21 = 44.07
Middle strip positive: 94.92 – 56.95 = 37.97
For Corner slab 15 ft*15 ft:
Mo = (1 / 8) Wl2ln² = (1 / 8) * 311.25 * 15 * {15 – (16 / 12)}² = 109 kip – ft.
Interior negative: 109 * 0.70 = 76.3
Exterior negative: 109 * 0.26 = 28.34
Exterior positive: 109 * 0.52 = 56.68
Exterior negative column strip = 28.34
Exterior negative middle strip = 0
Interior negative column strip = 0.75 * 76.3 = 57.23
Interior negative middle strip = 76.30 – 57.23 = 19.1
Interior positive column strip = 0.60 * 56.68 = 34
Interior positive middle strip = 56.68 – 34 = 22.67
ρ max = 0.85² * (3.5 / 60) * (0.003 / 0.003+0.004) = 0.018
As min = 0.0018 * 7.5 * 12 = 0.162 in²
20 ft direction ρmin = 0.162 / (6 * 12) = 0.00225
15 ft direction ρmin = 0.162 / (6.50 *12) = 0.00208
d² = Mo / [0.90 * 0.018 * 60000 *12{1 – (0.59 * 0.018 * 60 / 3.50)}]
d = √ (Mo / 9540.50)
d = √ (26.44*12000/9540.50)
d = 5.80 in.
For exterior middle slab 20 ft*15 ft:
Long direction:
Mo = [311.25 * 15 * (20-16 / 12) ²] / 8 = 203.35 ft-kip
Negative moment = 0.65 * 203.35 = 132.2 ft – kip
Positive moment = 0.35 * 203.35 = 71.2 ft – kip
Column strip negative moment: 0.75 * 132.20 = 99.15 ft – kip
Column strip positive moment: 0.60 * 71.20 = 42.7 ft – kip
Middle strip negative moment: 132.20 – 99.15 = 33.05 ft – kip
Middle strip positive moment: 71.20 – 42.70 = 28.5 ft – kip
Short direction:
Mo = [311.25 * 20 * (15 – 16 / 12)²] / 8 = 145.34 ft – kip
Interior negative moment = 0.70 * 145.34 = 101.74 ft – kip
Interior positive moment = 0.52*145.34 = 75.58 ft – kip
Exterior negative moment = 0.26 * 145.34 = 37.8 ft – kip
Exterior negative column strip: 37.80 * 1 = 37.8 ft – kip
Exterior negative middle strip: 0
Interior negative column strip: 101.74 * 0.75 = 76.31 ft-kip
Interior negative middle strip: 101.74 – 76.31 = 25.43 ft – kip
Positive column strip: 0.60 * 75.58 = 45.35 ft – kip
Positive middle strip: 0.40 * 75.58 = 30.23 ft – kip
The table below is showing reinforcement requirement for 15 ft *15 ft panel
Table 3.13: Design of slab reinforcement in 15 ft *15 ft panel
| Strip type | Moment type | Strip width b in. | Mu | |
Ft-kipEffective depth of the slab d inMu*12/b
The table below is showing reinforcement requirement for 20 ft * 20 ft panel
Table 3.14: Design of slab reinforcement in 20 ft *20 ft panel
| Strip type | Moment type | Strip width b in. | Mu |
Ft-kipEffective depth of the slab d inMu*12/b
Ft-kip/ft
Steel density ρRequired steel area As=ρbd in²
The table below is showing reinforcement requirement for 15 ft * 20 ft panel
Table 3.15: Design of slab reinforcement in 15 ft *20 ft panel
| Strip type | Moment type | Strip width b in. | Mu |
Ft-kipEffective depth of the slab d inMu*12/b
Ft-kip/ft
Steel density ρRequired steel area As=ρbd in²
The figures below is showing the reinforcement arrangement in slab in flat plate structure
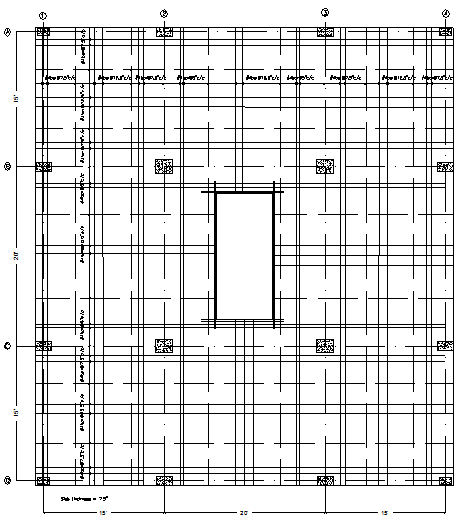
Figure 3.4: Reinforcement arrangement in slab in flat plate structure (long direction).
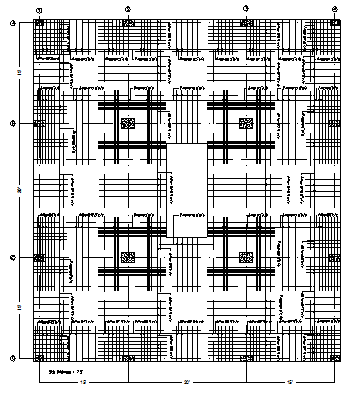
Figure 3.5: Reinforcement arrangement in slab in flat plate structure (short direction).
Punching Shear Check:
For Column- C6, C7, C11, C13:
Maximum spacing = 7.50 * 20 = 15 in
Vu = 0.31125 [17.50*17.5 – (22 * 22 / 144)] = 94.27 kip
D / 2 = 6 / 2 = 3 in
bo = 4 (16+6) = 88
Since, βc < 2;
φVc = φ 4√fc’ * bod = 0.75 * 4√3500 * 0.08 * 6.50 = 101.55 kip
For Column- C1, C4, C10, C16:
Vu = 0.31125 [8.20 * 8.20 – (19 * 19 / 144)] = 20.20 kip
bo = 19+19 = 38
φVc = φ 4√fc’ * bod = 0.75 * 4√3500 * 38 * 6.50 = 43.84 kip
For Column – C2, C3, C5, C8, C9, C12, C14, C15:
Vu = 0.31125 [17.50 * 8.20 – (19 * 22 / 144)] = 44 kip
bo = 19 * 20 + 22 = 60
φVc = φ 4√fc’ * bod = 69.22 kip]

Figure 3.6: Distribution of total static moment M0 to critical section for positive and negative bending.
Beam design
For beam design required data such as moment values, shears are taken from ETABS analysis report shown in table – and designed various beams for various floors of the beam supported structure.
Design of the beam: B1, B3, B4, B6, B7, B9, B10, B11 ( at 6th story )
From load combination:
Maximum moment:
End section:
Negative moment = 42.61 k-ft
Mu = ф ρfy bd² (1- 0.59 ρfy / fc)
d² = Mu / фρfy bd (1- 0.59 ρfy / fc)
ρ max = 0 .75 ρb, ρb = 0.85 β1 f’c / fy * 87000 / (87000 + 60000)
= 0.85 * 0.85 * 3.50 / 60 * 87000 / (87000 + 60000) = 0.02494
ρ max = 0.75 * 0.02494 = 0.0187
d² = 42.61 * 12 / 0.90 * 0.0187 * 60 *10 (1- 0.59*0.0187*60/3.50)
d = 7.90, Clear cover = 2”, Total depth = 7.90+2 = 9.90 say, d = 10”
Provided Beam size = 10” * 10”, d = 10”- 20”= 8”
Main steel calculation:
As = Mu / φfy (d – a / 2) = 42.61 * 12 / 0.9 * 60 (8 – 1 / 2)
= 1.262 in², a = Asfy / 0.85fc bw, a = 1.262 * 60 / 0.85 * 3.5 * 10 = 2.545 in.
As = 42.61 * 12 / 0.9 * 60 (8 – 2.545 / 2) = 1.407 in²
a = 1.407 * 60 / 0.85 * 3.50 * 10 = 2.837 in.
As = 42.61 * 12 / 0.9 * 60 (8 – 2.837 / 2) = 1.438 in²
Use – 2 # 7 +1 # 5 bars
Main steel calculation:
Mid section:
As = Mu / φfy (d – a / 2) = 30.30 * 12 / 0.9 * 60 (8 – 1 / 2)
= 0.897 in², a = Asfy / 0.85fc bw, a = 0.897 * 60 / 0.85 * 3.5 *10 = 1.809 in
As = 30.30 * 12 / 0.9 * 60 (8 – 1.809 / 2) = 0.949 in²
a = 0.949 * 60 / 0.85 * 3.5 * 10 = 1.91 in.
As = 30.30 * 12 / 0.90 * 60 (8 – 1.91 / 2) = 0.955 in² use – 3 # 5 bars
Shear reinforcement design:
V= Vu – φVc
= 16.21 – (2 * 0.85√3500 * 10 * 8) / 1000 = 8.164 kip
4 √fc bw d = (4√3500 * 10 * 8) / 1000 = 16.09 kip
Vs < 4√fc bw d So, ok
Stirrup spacing:
1) Smax = Avfy / 50 bw = (2 * 0.11 * 60000) / 50 * 10 = 26.40”
2) Smax = d / 2 = 8 / 2 = 4”
3) Smax = 24”
4) S = φAvfyd / Vs = 0.85 * 2 * 0.11 * 60000 * 8 / (8.164 * 1000) = 10.99”
Use stirrups # 3 bar @ 4” c/c
Design of the beam: B2, B5, B8, B12 ( at 6th story )
From load combination:
Maximum moment:
End section:
Negative moment = 80.00 k – ft
Mu = ф ρfy bd² (1- 0.59 ρfy / fc)
d² = Mu / фρfy bd (1 – 0.59 ρfy / f’c)
ρ max = 0.75 ρb, ρb = 0.85 β1 fc / fy * 87000 / (87000 + 60000)
= 0.85 * 0.85 * 3.5 / 60 * 87000 / (87000 + 60000) = 0.02494
ρ max = 0.75 * 0.02494 = 0.0187
d² = 80.0 * 12 / 0.90 * 0.0187 * 60 * 10 (1- 0.59 * 0.0187 * 60 / 3.50)
d = 10.82, Clear cover = 2”, Total depth = 10.82 + 2 = 12.82. Say d = 15
Provided Beam size = 15” * 10”, d = 15”- 2”= 13”
Main steel calculation:
As = Mu / φfy (d – a / 2)
= 80.0 *12 / 0.9 * 60 (13 – 1 / 2)
= 1.422 in², a = Asfy / 0.85fc bw, a = 1.422 * 60 / 0.85 * 3.5 * 10 = 2.868 in
As = 80.0 * 12 / 0.90 * 60 (8 – 2.868 / 2) = 1.537 in²
a = 1.537 * 60 / 0.85 * 3.50 * 10 = 3.099 in.
As = 80.0 * 12 / 0.90 * 60 (8 – 3.099 / 2) = 1.552 in², use – 2 # 7 +2 # 5 bars
Main steel calculation:
Mid section:
As = Mu / φfy (d – a / 2)
= 57.29 * 12 / 0.90 * 60 (13 – 1 / 2)
= 1.018 in², a = Asfy / 0.85fc bw, a = 1.018 * 60 / 0.85 * 3.5 *10 = 2.053 in
As = 57.29 * 12 / 0.90 * 60 (13 – 2.053 / 2) = 1.063 in²
a = 1.063 * 60 / 0.85 * 3.50 * 10 = 2.143 in.
As = 57.29 * 12 / 0.90 * 60 (13 – 2.143 / 2) = 1.067 in² use – 4 # 5 bars
Shear reinforcement design:
Vs = Vu – φVc
= 24.14 – (2 * 0.85√3500 * 10 *13) / 1000 = 11.06 kip
4 √fc bw d = (4√3500 * 10 * 13) / 1000 = 30.763 kip
Vs < 4√fc bw d So, ok
Stirrup spacing:
1) Smax = Avfy / 50 bw = (2 * 0.11* 60000) / 50 * 10 = 26.40”
2) Smax = d / 2 = 13 / 2 = 6½”
3) Smax = 24”
4) S = φ Avfy d / Vs = 0.85 * 2 * 0.11 * 60000 * 13 / (11.06 * 1000) =13.1”
Use stirrups # 3 bar @ 6½” c/c
Design of the beam: B14, B17, B20, B23 ( at 6th story )
From load combination:
Maximum moment at end section
Negative moment = 132.38 k – ft
Mu = ф ρfy bd² (1 – 0.59 ρfy / fc)
d² = Mu / (фρfy bd (1- 0.59 ρfy / fc)
ρ max = 0.75 ρb, ρb = 0.85 β1 fc / fy * 87000 / (87000 + 60000)
= 0.85 * 0.85 * 3.50 / 60 * 87000 / (87000 + 60000) = 0.02494
ρ max = 0.75 * 0.02494 = 0.0187
d² = 132.38 * 12 / 0.90 * 0.0187 * 60 * 10 (1-0.59 * 0.0187 * 60 / 3.50)
d = 13.92, Clear cover = 2”, Total depth = 13.92 + 2 = 15.92.92. Say, d = 18”
Provided Beam size = 18” * 10”, d = 18”- 2”= 16”
Main steel calculation:
As = Mu / φfy (d – a / 2)
= 132.38 * 12 / 0.90 * 60 (16 – 1 / 2)
= 1.897 in², a = Asfy / 0.85fc bw, a = 1.897 * 60 / 0.85 * 3.5 * 10 = 3.825 in
As = 132.38 * 12 / 0.90 * 6 (16 – 3.825 / 2) = 2.088 in²
a = 2.088 * 60 / 0.85 * 3.50 * 10 = 4.211 in.
As = 132.38 * 12 / 0.90 * 60 (16 – 4.211 / 2) = 2.117 in² use – 2 # 8 +2 # 5 bars
Main steel calculation:
Mid section:
As = Mu / φfy (d – a / 2)
= 103.96 * 12 / 0.90 * 60 (16 – 1 / 2)
= 1.49 in a = Asfy / 0.85fc, bw a = 1.49 * 60 / 0.85 * 3.5 *10 = 3.00 in.
As = 103.96 * 12 / 0.90 * 60 (16 – 3.00 / 2) = 1.593 in²
a = 1.593 * 60 / 0.85 * 3.50 * 10 = 3.217 in.
As = 103.96 *12 / 0.90 * 60 (16 – 3.217 / 2) = 1.605 in². Use – 2 # 6 +2 # 5 bars
Shear reinforcement design:
Vs = Vu – φVc
= 40.84 – (2 * 0.85√3500 * 10 * 16) / 1000 = 24.78 kip
4 √fc bw d = (4√3500 * 10 * 16) / 10 00 = 32.183 kip
Vs < 4√fc bw d. So, ok
Stirrup spacing:
1) Smax = Avfy / 50 bw = (2 * 0.11 * 60000) / 50 * 10 = 26.40”
2) Smax = d / 2 = 16 / 2 = 8”
3) Smax = 24”
4) S = φAvfy d / Vs = 0.85 * 2 * 0.11* 60000 *16 / (24.78 * 1000) = 7.24 ”
Use stirrups # 3 bar @ 7” c/c
Design of the beam: B13, B15, B16, B18, B19, B21, B22, B24 (at 6th story)
From load combination:
Maximum moment at end section
Negative moment = 64.59 k – ft
Mu = ф ρfy bd² (1- 0.59 ρfy / fc)
d² = Mu / (ф ρfy bd (1 – 0.59 ρfy / fc)
ρ max = 0 .75 ρb, ρb = 0.85 β1 fc / fy * 87000 / (87000 + 60000)
= 0.85 * 0.85 * 3.5 / 60 * 87000 / (87000 + 60000) = 0.02494
ρ max = 0.75 * 0.02494 = 0.0187
d² = 64.59 * 12 / 0.90 * 0.0187 * 60 * 10 (1- 0.59 * 0.018 * 60 / 3.50)
d = 9.72, Clear cover = 2”, Total depth = 9.72+2= 11.72. Say, d = 12”
Provided Beam size = 12” * 10”, d = 12”- 2” = 10”
Main steel calculation:
As = Mu / φfy (d – a / 2)
= 64.59 * 12 / 0.90 * 60 (10 – 1 / 2) = 1.51 in², a = Asfy / 0.85fc bw
a = 1.51 * 60 / 0.85 * 3.50 * 10 = 3.045 in
As = 64.59 * 12 / 0.90 * 60 (10 – 3.045 / 2) = 1.693 in²
a = 1.693 * 60 / 0.85 * 3.5 * 10 = 3.414 in.
As = 64.59 *12 / 0.90 * 60 (10 – 3.414 / 2) = 1.73 in² use – 2 # 7 +2 # 5 bars
Main steel calculation:
Mid section:
As = Mu / φfy (d – a / 2)
= 51.98 * 12 / 0.90 * 60 (10 – 1 / 2)
= 1.215 in², a = Asfy / 0.85fc, bw a = 1.215 * 60 / 0.85 * 3.5 * 10 = 2.450 in
As = 51.98*12 / 0.90 * 60 (10 – 2.45 / 2) = 1.316 in²
a = 1.316 * 60 / 0.85 * 3.50 * 10 = 2.609 in.
As = 51.98 * 12 / 0.90 * 60 (10 – 2.609 / 2) = 1.328 in² use – 2 # 6 +2 # 5 bars
Shear reinforcement design:
Vs = Vu – φVc
= 25.40 – (2 * 0.85√3500 * 10 * 10) / 1000 = 15.34 kip
4 √fc bw d = (4√3500 * 10 * 10) / 1000 = 23.66 kip
Vs < 4√fc bw d So, ok
Stirrup spacing:
1) Smax = Avfy / 50 bw = (2 * 0.11 * 60000) / 50 * 10 = 26.40”
2) Smax = d / 2 = 10 / 2 = 5”
3) Smax = 24”
4) S = φAvfy d / Vs = 0.85 * 2 * 0.1 1* 60000 * 10 / (15.34 * 1000) = 7.31 ”
Use stirrups # 3 bar @ 5” c/c
Design of the beam: B1, B3, B4, B6, B7, B9, B10, B11 ( at 5th story )
From load combination:
Maximum moment at end section
Negative moment = 42.61 k – ft
Mu = ф ρfy bd² (1 – 0.59 ρfy / fc)
d² = Mu / ф ρfy bd (1- 0.59 ρfy / fc)
ρ max = 0.75 ρb ,
ρb = 0.85 β1 fc / fy * 87000 / (87000 + 60000)
= 0.85 * 0.85 * 3.5 / 60 * 87000 / (87000 + 60000) = 0.02494
ρmax = 0.75 * 0.02494 = 0.0187
d² = 42.61 * 12 / 0.90 * 0.0187 * 60 * 10 (1- 0.59 * 0.0187 * 60/3.50)
d = 7.90, Clear cover = 2”, Total depth = 7.90 + 2 = 9.90. Say, d = 10”
Provided Beam size = 10” * 10”, d = 10”- 2”= 8”
Main steel calculation:
As = Mu / φfy (d – a / 2)
= 42.61 * 12 / 0.90 * 60 (8 – 1 / 2) = 1.262 in², a = Asfy / 0.85fc bw,
a = 1.262 * 60 / 0.85 * 3.50 * 10 = 2.545 in
As = 42.61 * 12 / 0.90 * 60 (8 – 2.545 / 2)
As = 1.407 in²
a = 1.407 * 60 / 0.85 * 3.50 * 10 = 2.837 in.
As = 42.61 * 12 / 0.90 * 60 (8 – 2.837 / 2) = 1.438 in². Use – 2 # 7 +1 # 5 bars
Main steel calculation:
Mid section:
As = Mu / φfy (d – a / 2)
= 30.30 * 12 / 0.90 * 60 (8 – 1 / 2) = 0.897 in², a = Asfy / 0.85fc bw,
a = 0.897 * 60 / 0.85 * 3.50 * 10 =1.809 in.
As = 30.30 * 12 / 0.9 * 60 (8 – 1.809 / 2) = 0.949 in²
a = 0.949 * 60 / 0.85 * 3.50 * 10 = 1.91 in.
As = 30.30 * 12 / 0.90 * 60 (8 – 1.91 / 2) = 0.955 in². Use – 3 # 5 bars
Shear reinforcement design:
Vs = Vu – φVc
= 16.21 – (2 * 0.85√3500 * 10 * 8) / 1000 = 8.164 kip
4φ √fc bw d = (4 * 0.85√3500 *10 * 8) / 1000 = 16.09 kip
Vs < 4√fc bw d So, ok
Stirrup spacing:
1) Smax = Avfy / 50 bw = (2*.11*60000) / 50 * 10 = 26.40”
2) Smax = d / 2 = 8 / 2 = 4”
3) Smax = 24”
4) S = φ Avfy d / Vs = 0.85 * 2 * 0.11 * 60000 * 8 / (8.164 * 1000) = 10.99”
Use stirrups # 3 bar @ 4” c/c
Design of the beam: B2, B5, B8, B12 ( at 5th story )
From load combination:
Maximum moment:
End section:
Negative moment = 80.00 k – ft
Mu = ф ρfy bd² (1- 0.59 ρfy / fc)
d² = Mu / ф ρfy bd (1- 0.59 ρfy / fc)
ρ max = 0.75 ρb , ρb = 0.85 β1 fc / fy * 87000 / (87000 + 60000)
= 0.85 * 0.85 * 3.50 / 60 * 87000 / (87000 + 60000) = 0.02494
ρ max = 0.75 * 0.02494 = 0.0187
d² = 80.0 * 12 / 0.90 * 0.0187 * 60 * 10 (1- 0.59 * 0.0187 * 60 / 3.50)
d = 10.82, Clear cover = 2”, Total depth = 10.82 + 2 = 12.82. Say, d = 15
Provided Beam size = 15” * 10”, d = 15”- 2”= 13”
Main steel calculation:
As = Mu / φfy (d – a / 2)
= 80.0 * 12 / 0.90 * 60 (13 – 1 / 2)
= 1.422 in², a = Asfy / 0.85fc bw,
a = 1.422 * 60 / 0.85 * 3.50 * 10 = 2.868 in
As = 80.0 * 12 / 0.90 * 60 (8 – 2.868 / 2) = 1.537 in²
a = 1.537 * 60 / 0.85 * 3.50 * 10 = 3.099 in.
As = 80.0 * 12 / 0.90 * 60 (8 – 3.099 / 2) = 1.552 in². Use – 2 # 7 +2 # 5 bars
Main steel calculation:
Mid section:
As = Mu / φfy (d – a / 2)
= 57.29 * 12 / 0.90 * 60 (13 – 1 / 2) = 1.018 in², a = Asfy / 0.85fc bw,
a = 1.018 * 60 / 0.85 * 3.5*10 = 2.053 in.
As = 57.29 * 12 / 0.90 * 60 (13- 2.053 / 2) = 1.063 in²
a = 1.063 * 60 / 0.85 * 3.50 * 10 = 2.143 in.
As = 57.29 * 12 / 0.90 * 60 (13 – 2.143 / 2) = 1.067 in². Use – 4 # 5 bars
Shear reinforcement design:
Vs = Vu – φVc
= 24.14 – (2 * 0.85√3500 * 10 * 13) / 1000 = 11.06 kip
4φ √fc bw d = (4 * 0.85√3500 * 10 * 13 / 1000 = 30.763 kip
Vs < 4√fc bw d So, ok
Stirrup spacing:
1) Smax = Avfy / 50 bw = (2 * 0.11 * 60000) / 50 * 10 = 26.40”
2) Smax = d / 2 = 13 / 2 = 6½”
3) Smax = 24”
4) S = φ Avfy d / Vs = 0.85 * 2 * 0.11 * 60000 * 13 / (11.06 * 1000) = 13.1”
Use stirrups # 3 bar @ 6½” c/c
Design of the beam: B14, B17, B20, B23 ( at 5th story )
From load combination:
Maximum moment at end section
Negative moment = 132.38 k-ft
Mu = ф ρfy bd² (1 – 0.59 ρfy / f’c)
d² = Mu / (ф ρfy bd (1- 0.59 ρfy / fc)
ρ max = 0.75 ρb, ρb = 0.85 β1 f’c / fy * 87000 / (87000 + 60000)
= 0.85 * 0.85 * 3.5 / 60 * 87000 / (87000 + 60000) = 0.02494
ρ max = 0.75 * 0.02494 = 0.0187
d² = 132.38 * 12 / 0.90 * 0.0187 * 60 * 10 (1- 0.59 * 0.0187 * 60 / 3.50)
= 13.92, Clear cover = 2”, Total depth = 13.92 + 2 = 15.92. Say, d = 18”
Provided Beam size = 18” * 10”, d = 18”- 2”= 16”
Main steel calculation:
As = Mu / φfy (d – a / 2)
= 132.38 * 12 / 0.90 * 60 (16 – 1 / 2)
= 1.897 in², a = Asfy / 0.85fc bw, a = 1.897 * 60 / 0.85 * 3.5 * 10 = 3.825 in.
As = 132.38 * 12 / 0.90 * 60 (16 – 3.825 / 2) = 2.088 in²
a = 2.088 * 60 / 0.85 * 3.50 * 10 = 4.211 in.
As = 132.38 * 12 / 0.90 * 60 (16 – 4.211 / 2) = 2.117 in². Use – 2 # 7 +3 # 5 bars
Main steel calculation:
Mid section:
As = Mu / φfy (d – a / 2)
= 103.96 * 12 / 0.90 * 60 (16 – 1 / 2)
= 1.49 in², a = Asfy / 0.85fc bw, a = 1.49 * 60 / 0.85 * 3.5*10 = 3.00 in.
As = 103.96 * 12 / 0.90 * 60 (16 -3.00 / 2) = 1.593 in²
a = 1.593 * 60 / 0.85 * 3.50 * 10 = 3.217 in.
As = 103.96 * 12 / 0.90 * 60 (16 – 3.217 / 2) = 1.605 in². Use – 4 # 6 bars
Shear reinforcement design:
Vs = Vu – φVc
= 40.84 – (2 * 0.85√3500 * 10 * 16) / 1000 = 24.78 kip
4 √fc bw d = (4√3500 * 10 * 16) / 1000 = 32.183 kip
Vs < 4√fc bw d So, ok
Stirrup spacing:
1) Smax = Avfy / 50 bw = (2 * 0.11 * 60000) / 50 * 10 = 26.40”
2) Smax = d / 2 = 16 / 2 = 8”
3) Smax = 24”
4) S = φ Avfy d / Vs= 0.85 * 2 * 0.11 * 60000 * 16 / (24.78 * 1000) = 7.24”
Use stirrups # 3 bar @ 7” c/c
Design of the beam: B13, B15, B16, B18, B19, B21, B22, B24 (at 4th story)
From load combination:
Maximum moment at end section
Negative moment = 64.59 k – ft
Mu = ф ρfy bd² (1- 0.59 ρfy / f’c)
d² = Mu / (фρfy bd (1- 0.59 ρf / f’c)
ρ max = 0.75 ρb, ρb = 0.85 β1 f’c / fy * 87000 / (87000 + 60000)
= 0.85 * 0.85 * 3.5 / 60 * 87000 / (87000 + 60000) = 0.02494
ρ max = 0.75 * 0.02494 = 0.0187
d² = 64.59 * 12 / 0.90 * 0.0187 * 60 * 10 (1-0.59 * 0.0187 * 60 / 3.50)
d = 9.72, Clear cover = 2”, Total depth = 9.72 + 2= 11.72. Say, d = 12”
Provided Beam size = 12” * 10”, d = 12”- 2”= 10”
Main steel calculation:
As = Mu / φfy (d – a / 2)
= 64.59 * 12 / 0.90 * 60 (10 – 1 / 2) = 1.51 in², a = Asfy / 0.85f’c bw
a = 1.51 * 60 / 0.85 * 3.50 * 10 = 3.045
As = 64.59 * 12 / 0.90 * 60 (10 – 3.045 / 2) = 1.693 in²
a = 1.693 * 60 / 0.85 * 3.5 * 10 = 3.414 in.
As = 64.59 * 12 / 0.90 * 60 (10 – 3.414 / 2) = 1.73 in². Use – 2 # 7 +2 # 5 bars
Main steel calculation:
Mid section:
As = Mu / φfy (d – a / 2)
= 51.98 * 12 / 0.90 * 60 (10 – 1 / 2) = 1.215 in², a = Asfy / 0.85f’c bw
a = 1.215 * 60 / 0.85 * 3.50 * 10 = 2.450 in.
As = 51.98 * 12 / 0.90 * 60 (10 – 2.45 / 2) = 1.316 in²
a = 1.316 * 60 / 0.85 * 3.50 * 10 = 2.609 in.
As = 51.98 * 12 / 0.90 * 60 (10 – 2.609 / 2) = 1.328 in². Use – 2 # 6 +2 # 5 bars
Shear reinforcement design:
Vs = Vu – φVc
= 25.40 – (2 * 0.85√3500 * 10 * 10) / 1000 = 15.34 kip
4 √fc bw d = (4√3500 * 10 * 10) / 1000 = 23.66 kip
Vs < 4√fc bw d So, ok
Stirrup spacing:
1) Smax = Avfy / 50 bw = (2 * 0.11 * 60000) / 50 * 10 = 26.40”
2) Smax = d / 2 = 10 / 2 = 5”
3) Smax = 24”
4) S = φ Avfy d / Vs = 0.85 * 2 * 0.11 * 60000 * 10 / (15.34*1000) = 7.31 ”
Use stirrups # 3 bar @ 5” c/c
Design of the beam: B1, B3,B4, B6, B7, B9, B10, B11 ( at 4th story )
From load combination:
Maximum moment:
End section:
Negative moment = 87.25 k – ft
Mu = ф ρfy bd² (1- 0.59 ρfy / fc)
d² = Mu/ фρfy bd (1- 0.59 ρfy / fc)
ρ max = 0 .75 ρb, ρb = 0.85 β1 f’c / fy * 87000 / (87000 + 60000)
= 0.85 * 0.85 * 3.5 / 60 * 87000 / (87000 + 60000) = 0.02494
ρ max = 0.75 * 0.02494 = 0.0187
d² = 87.25 * 12 / 0.90 * 0.0187 * 60 * 10 (1- 0.59 * 0.0187 * 60 / 3.50)
d = 11.29, Clear cover = 2”, Total depth = 11.29 + 2= 13.29. Say, d = 15”
Provided Beam size = 15” * 10”, d = 15”- 2”= 13”
Main steel calculation:
As = Mu / φfy (d – a / 2)
= 87.25 * 12 / 0.90 * 60 (13 – 1 / 2) = 1.551 in², a = Asfy / 0.85fc bw
a = 1.551 * 60 / 0.85 * 3.50 * 10 = 3.128 in.
As = 87.25 * 12/0.90 * 60 (13 – 3.128 / 2) = 1.695 in²
a = 1.695 * 60 / 0.85 * 3.50 * 10= 3.418 in.
As = 87.25 * 12 / 0.90 * 60 (10 – 3.418 / 2) = 1.717 in². Use – 2 # 7 + 2 # 5 bars
Main steel calculation:
Mid section:
As = Mu / φfy (d – a / 2)
= 35.0 * 12 / 0.90 * 60 (13 – 1 / 2) = 0.622 in², a = Asfy / 0.85f’c bw
a = 0.622 * 60 / 0.85 * 3.50 * 10 = 1.254 in.
As = 35.00 * 12 / 0.90 * 60 (13 – 1.254 / 2) = 0.628 in²
a = 0.628 * 60 / 0.85 * 3.50 * 10 = 1.266 in.
As = 35.0 * 12 / 0.90 * 60 (13 – 1.266/2) = 0.63 in². Use – 2 # 6 bars
Shear reinforcement design:
Vs = Vu – φVc
= 22.23 – (2 * 0.85√3500 * 10 * 13) / 1000 = 9.15 kip
4 √fc bw d = (4√3500 * 10 * 13) /1000 = 30.76 kip
Vs < 4√fc bw d So, ok
Stirrup spacing:
1) Smax = Avfy / 50 bw = (2 * 0.11* 60000) / 50 * 10 = 26.40”
2) Smax = d / 2 = 13 / 2 = 6½”
3) Sma = 24”
4) S = φ Avfy d / Vs = 0.85 * 2 * 0.11 * 60000 * 13 / (9.15 * 1000) = 15.94”
Use stirrups # 3 bar @ 6½” c/c
Design of the beam: B2, B5, B8, B12 ( at 4th story )
From load combination:
Maximum moment:
End section:
Negative moment = 108.93 k-ft
Mu = ф ρfy bd² (1- 0.59 ρfy / fc)
d² = Mu / фρfy bd (1- 0.59 ρfy / fc)
ρ max = 0.75 ρb, ρb = 0.85 β1 fc / fy * 87000 / (87000 + 60000)
= 0.85 * 0.85 * 3.5 / 60 * 87000 / (87000 + 60000) = 0.02494
ρ max = 0.75 * 0.02494 = 0.0187
d² = 108.93 * 12 / 0.90 * 0.0187 * 60 * 10(1- 0.59 * 0.0187 * 60 / 3.50)
d = 12.63, Clear cover = 2”, Total depth = 12.63 + 2 = 14.63. Say, d = 15”
Provided Beam size = 15” * 10”, d = 15”- 2”= 13”
Main steel calculation:
As = Mu / φfy (d – a / 2)
= 108.93 * 12 / 0.90 * 60 (13 – 1 / 2) = 1.936 in², a = Asfy / 0.85fc bw,
a = 1.936 * 60 / 0.85 * 3.50 * 10 = 3.904 in.
As = 108.93 * 12 / 0.90 * 60 (13 – 3.904 / 2) = 1.867 in²
a = 1.897 * 60 / 0.85 * 3.50 * 10 = 3.765 in.
As = 108.93 * 12 / 0.90 * 60 (13 – 3.765 / 2) = 2.177 in². Use – 2 # 8 +2 # 5 bars
Main steel calculation:
Mid section:
As = Mu / φfy (d – a / 2)
= 59.56 * 12 / 0.90 * 60 (13 – 1 / 2) = 1.058 in², a = Asfy / 0.85fc bw,
a = 1.058 * 60 / 0.85 * 3.50 * 10 = 2.133 in.
As = 59.56 * 12 / 0.90 * 60 (13 – 2.133 / 2) = 1.108 in²
a = 1.108 * 60 / 0.85 * 3.5 * 10 = 2.234 in.
As = 59.56 * 12 / 0.90 * 60 (13 – 2.234 / 2) = 1.113in². Use – 4 # 5 bars
Shear reinforcement design:
Vs = Vu – φVc
= 26.90 – (2 * 0.85√3500 * 10 * 13) / 1000 = 13.825 kip
4 √fc bw d = (4√3500 * 10 * 13) / 1000 = 30.763 kip
Vs < 4√fc bw d So, ok
Stirrups Spacing:
1) Smax = Avfy / 50 bw = (2 * 0.11 * 60000) / 50 * 10 = 26.40”
2) Smax = d / 2 = 13 / 2 = 6½”
3) Smax = 24”
4) S = φ Avfyd / Vs = 0.85 * 2 * 0.11 * 60000 * 13 / (13.825 * 1000) = 10.55”
Use stirrups # 3 bar @ 6½” c/c
Design of the beam: B14, B17, B20, B23 (at 4th story)
From load combination:
Maximum Moment:
End section:
Negative moment = 164.32 k-ft
Mu = ф ρfy bd² (1 – 0.59 ρfy / fc)
d² = Mu / (ф ρfy bd (1- 0.59 ρfy / fc)
ρ max = 0.75 ρb, ρb = 0.85 β1 fc / fy * 87000 / (87000 + 60000)
= 0.85 * 0.85 * 3.5 / 60 * 87000 / (87000 + 60000) = 0.02494
ρmax = 0.75 * 0.02494 = 0.0187
d² = 164.32 *12 / 0.90 * 0.0187 * 60 * 12 (1 – 0.59 * 0.0187 * 60 / 3.50)
d = 14.16, Clear cover = 2”, Total depth = 14.16 + 2 = 16.16. Say, d = 18”
Provided Beam size = 18” * 10”, d = 18”- 2”= 16”
Main steel calculation:
As = Mu / φfy (d – a / 2)
= 164.32 * 1 / 0.90 * 60 (16 – 1 / 2) = 2.355 in², a = Asfy / 0.85fc bw,
a = 2.355 * 60 / 0.85 * 3.50 * 12 = 3.957 in.
As = 164.32 * 12 / 0.90 * 60 (16 – 3.597 / 2) = 2.604 in²
a = 2.604 * 60 / 0.85 * 3.50 * 10 = 4.376 in.
As = 164.32 * 12 / 0.90 * 60 (16 – 4.376 / 2) = 2.643 in². Use – 2 # 9 +2 # 5 bars
Main steel calculation:
Mid section:
As = Mu / φfy (d – a / 2)
= 103.66 * 12 / 0.90 * 60 (16 – 1 / 2) = 1.49 in², a = Asfy / 0.85fc bw,
a = 1.49 * 60 / 0.85 * 3.5 * 10 = 3.00 in.
As = 103.66 * 12 / 0.90 * 60 (16 – 3.00 / 2) = 1.59 in²
a = 1.59 * 60 / 0.85 * 3.50 * 10 = 3.206 in.
As = 103.66 * 12 / 0.90 * 60 (16 – 3.206 / 2) = 1.60 in². Use – 2 # 7 +2 # 5 bars
Some are parts:
Analysis Between a Beam Supported Structure and a Flat Plate Structure (Part 1)
Analysis Between a Beam Supported Structure and a Flat Plate Structure (Part 2)
Analysis Between a Beam Supported Structure and a Flat Plate Structure (Part 3)